14+ Fakten über Relation Reflexiv Symmetrisch Transitiv Beispiele! Reflexive, symmetric and transitive relations© copyright 2017, neha agrawal.
Relation Reflexiv Symmetrisch Transitiv Beispiele | Then the relation is called transitive. If you want a tutorial, there's one here: Consider the set i of the integers and a relation be defined as arb if both a and b are odd. Äquivalenzrelationen sind für die mathematik und für die logik von großer bedeutung. If you want examples, great.
This is an example from a class. Reflexive symmetric & transitive relation examplewatch more videos at: Greater than(x>y)= reflexive, symmetric, or transitive the relation greater than is not reflexive as 5>5 is clearly false. Given a set of nodes representing the elements of our collection, a directed arrow from node x to node y represents the participation of (x,y) in a relation r. It is given by r:
It is given by r: Relations and functions || reflexive relation || symmetric relation || transitive relation || equivalence relations || part(2)#ahsec#cbsc#ncert The statement x > 5 which is true if x = 7 and false if x = 3. A →b such that r = a x b (⊆ a x b) is a universal relation. What is an equivalence relation? A ≤ b 3 define on r is neither reflexive nor symmetric nor transitive. Difference between reflexive and identity relation. Discuss the following relations for reflexivity, symmetricity and transitivity: Reflexive, symmetric, transitive and equivalence relations. Given a set of nodes representing the elements of our collection, a directed arrow from node x to node y represents the participation of (x,y) in a relation r. Reflexive means a r a for every a. There are eight relations on s = { a, b, c } that are reflexive and symmetric. Then the relation is called transitive.
For a relation to be an equivalence relation we need that it is reflexive, symmetric and transitive. Universal relation from a →b is reflexive, symmetric and transitive. Consider the set i of the integers and a relation be defined as arb if both a and b are odd. Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here. All rights reserved.you can now follow me on fa.
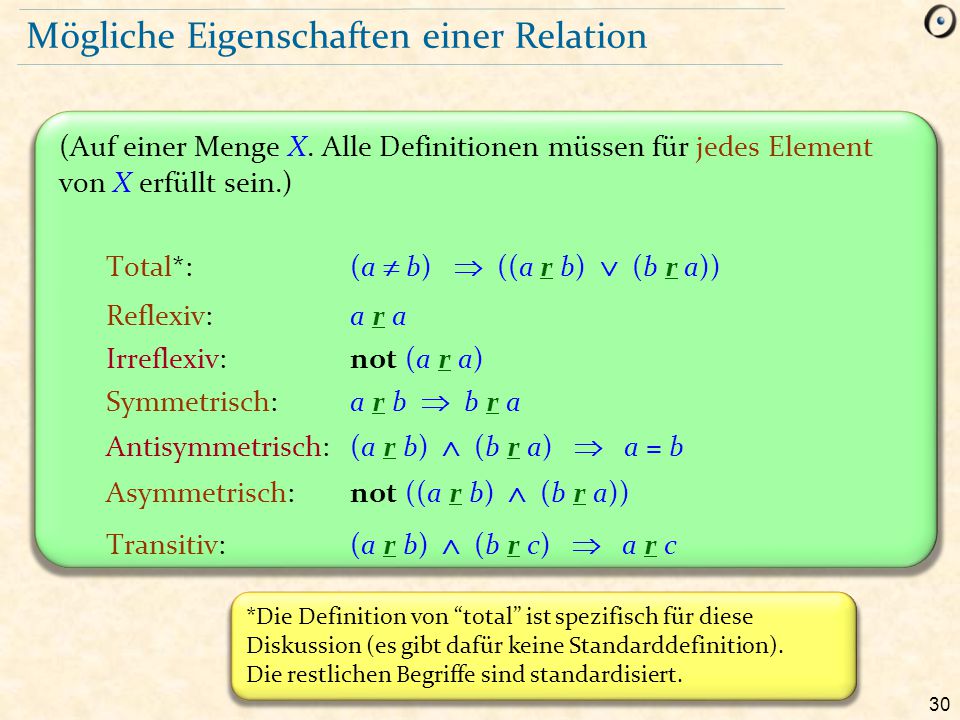
Cs40 reflexive symmetric transitive quiz select the number of questions per quiz: The relation r defined by lrm if l is perpendicular to m. The relation r is defined in n × n as follows (a, b) r (c, d) ⇔ a + d = b + c then r is view solution show that r = ( a , b ) : 1 let t be the set of all triangles in a plane with r a relation in t. A →b such that r = ∅ (⊆ a x b) is a null relation. Äquivalenzrelationen sind für die mathematik und für die logik von großer bedeutung. We look at three types of such relations: Given a set of nodes representing the elements of our collection, a directed arrow from node x to node y represents the participation of (x,y) in a relation r. Greater than(x>y)= reflexive, symmetric, or transitive the relation greater than is not reflexive as 5>5 is clearly false. A homogeneous relation r over a set x may be identified with a directed. But clearly sums are the same for a and a, so it is reflexive. Which is (i) symmetric but neither reflexive nor transitive.(ii) transitive but neither reflexive nor symmetric.(iii) reflexive and symmetric but not transitive.(iv) reflexive and transitive but not symmetric.(v) symmetric and transitive but not reflexive. 3x − y = 0} r = {(x, y):
We call a relation that is reflexive, symmetric, and transitive an equivalence relation. Determine the above relation is reflexive, symmetric and transitive. (i)relation r in the set a = {1, 2, 3…13, 14} defined as r = {(x, y): Eine äquivalenzrelation teilt eine menge restlos in disjunkte (elementfremde) untermengen, äquivalenzklassen genannt. A →b such that r = ∅ (⊆ a x b) is a null relation.
A →b such that r = ∅ (⊆ a x b) is a null relation. Unter einer äquivalenzrelation versteht man in der mathematik eine zweistellige relation, die reflexiv, symmetrisch und transitiv ist. A binary relation on s is a subset of the cartesian product s × s of ordered pairs of elements of s. Determine the above relation is reflexive, symmetric and transitive. Given a set of nodes representing the elements of our collection, a directed arrow from node x to node y represents the participation of (x,y) in a relation r. We call a relation that is reflexive, symmetric, and transitive an equivalence relation. Cs40 reflexive symmetric transitive quiz select the number of questions per quiz: In mathematics, a homogeneous relation (also called endorelation) over a set x is a binary relation over x and itself, i.e. It is not symmetric, since if 3>1, then 1>3 is false. 3x − y = 0} r = {(x, y): What is an equivalence relation? Let p denote the set of all straight lines in a plane. So let us check these if $ \equiv_5 $ is an equivalence relation.
Relation Reflexiv Symmetrisch Transitiv Beispiele: It is also simply called a (binary) relation over x.an example of a homogeneous relation is the relation of kinship, where the relation is over people.
0 Response to "14+ Fakten über Relation Reflexiv Symmetrisch Transitiv Beispiele! Reflexive, symmetric and transitive relations© copyright 2017, neha agrawal."
Post a Comment